Babylonian numerals can also be used for representing fractions. The idea is similar to the use of "decimal point" in decimal number system. For example, consider the decimal number "3.27". What are the place values of "2" and "7"?
| 2 means that there are 2 of " |
|
" |
| 7 means that there are 7 of " |
|
" |
| Therefore, 1.27 = 3 × 1 + 2 × |
|
+ 7 × |
|
For Babylonian number system, there is also a concept of "sexagesimal point" which separates the integral and fractional part of any Babylonian numbers.
Example 2.5.
We use ";" to denote the sexagesimal point. Let's consider the following examples:
1. What is the value of "21 ; 3 45"?
| 1. 21 × 1 + 3 × |
|
+ 45 × |
|
= 21 |
|
2. What is the value of "2 26 ; 23 6"?
| 2. 2 × 601 + 26 × 1 + 23 × |
|
+ 6 × |
|
= 146 |
|
However, there was no symbol for sexagesimal point in ancient Babylonian and this really caused lots of confusions since sometimes it is very hard to determine the fractional part of a Babylonian number just by looking into the context:
Example 2.6.
Consider the following example:
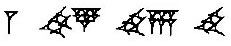
Credit: Queen’s University, Math@Home 2001-2002
What are its possible values?
1 57 46 40, 1 57 46 ; 40, 1 57 ; 46 40, 1 ; 57 46 40 and ; 1 57 46 40.